
We went camping recently and on the way back home we stopped at the top of the mountain pass to see some amazing mountain ranges and catch a glimpse of our favorite volcano. (Lol, it’s not erupting — thankfully! — but that’s what our 4 year old always calls it.)
N (the 4 year old) has a delightful habit of picking up rocks everywhere he goes for his “rock collection.” (question: what does he think this is? Does he envision a huge pile of rocks in our house that he collects from his various trips? Is he secretly squirreling them all away somewhere? He’s pretty sure this is a thing, and I’m pretty sure it isn’t. … Time will tell, I suppose.)
So we were at the top of the mountain and N picked up a handful of rocks, per usual, and asked if he could throw them down the mountain. He and lil Dude love throwing rocks in water, so this seemed like a reasonable request — he was quite surprised when i said no. Actually it came out more like “NowayNEVERDOTHATwhatdoyouthinkyou’redoing?!” as terrible visions of rock slides swarmed up in my ever-anxious/protective mama brain.
He stopped, mid wind-up, and looked confused. I tried to explain the basic idea of a rock slide and it turned into a pretty neat math conversation, (although I have reason to fear the actual topic).
On the ride back home we talked about how if he throws one rock down the mountain it might knock loose two more rocks, which isn’t really a problem — except that each of those rocks might knock two more rocks (to keep the math simple) and each of those rocks might knock two more rocks each, and pretty quickly all the rocks are falling down the mountain. N looked properly terrified.
It’s kind of a fun tongue-twister too:
If one rock knocks two rocks, and two rocks knock four, and four rock-knocking rocks knock loose eight rocks, how many rocks will be knocked next?
or something like that.
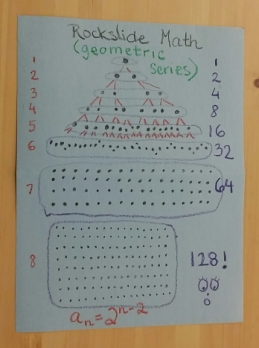
He counted up to 16 and was impressed enough by the grandeur of the situation so we stopped there. But for the record, the first 10 numbers in the series would be:
1, 2, 4, 8, 16, 32, 64, 128, 256, 512 …
and you get the idea how fast things can get out of hand when a number is doubled every time to create the next number in a series.
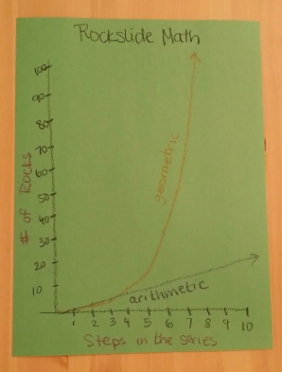
Later I was still thinking about this and wondering if this was an example of exponential growth. I drew some diagrams to show N the difference between simply adding 2 to each term in a series (every second after the first rock falls, two additional rocks fall down … or there might be a better way to explain that?) — this would be an arithmetic series — and saying that every rock that falls knocks 2 rocks down (essentially doubling the amount of rocks falling each time) — this is a geometric series. This is admittedly pretty fuzzy math, because you wouldn’t actually have clear intervals to measure the different “groups” of falling rocks, and every single rock wouldn’t actually knock exactly two more, and technically exponential growth that occurs naturally is based on “e,” a constant value that is related to the inverse of the natural logarithmic function (might be missing something here) … etc. But I figured it was a good enough approximation to a complex mathematical idea … and hopefully the exposure to the thought of increasing patterns of numbers in a series (sequence?) is a valuable learning experience. At the very least, I think we’ve all learned not to throw rocks down the mountain! 🙂
And I realized how long it’s been since I learned about all this and how quickly it dissipates. Also, you can learn pretty much anything you want to know on the internet, so really … why go to school? lol … kind of …

Somewhere in there I remembered a song I had run across when I was doing my math major years ago — it’s a song that a college professor (Alan AtKisson) wrote to explain the concept of exponential growth to his students and for some silly reason I loved it and remembered it all these years later. This is the link to the youtube video of him singing it in front of his class. (He has several other songs about other economic principles as well.) So for the past week or so, we all (even lil Dude) have been breaking into song randomly with the refrain “Exponential growth, oh, it’s exponential growth; yes it’s creeping up behind you, yes, it’s exponential growth!”

As I was writing this post, I remembered that we have a book about this concept that does a great job explaining it in a fun, easy-to-understand way for kids with cute illustrations. It’s called 512 Ants on Sullivan Street (by
Note: None of these are affiliate links. I’m just providing links for things that I’ve found interesting or helpful or entertaining, in case any of it is relevant to you as well. 🙂
Signing off with another pretty picture of the river by our campsite (very safe for rock-throwing exercises!)

Other Math Monday posts:
- Ten-frame math with seashells
- Cotton Ball Algebra
- Sticker Bar Graphs
- Tessellations
- RightStart curriculum
Math Resources for Teachers, Tutors, and Homeschooling Families:
If you’d like to see my own growing library of educational resources (mostly focused on math) for pre-K through high school students, you can visit my online store at: https://www.teacherspayteachers.com/Store/Sandra-Balisky. You can read more about my new venture in this blog post here.




6 comments on “Math Monday: Exponential growth (for young kids)”